Table of Content
1) Introduction
2) Basic Problem on Age question
3) Advance Level Questions
4) Shortcut tricks ratio question
5) Shortcut trick videos
6) Shortcut trick using option method
Problem on ages questions can be identified on each and every competitive exams. Let it be a government exam or any other competitive exam. There is a high chance of occurrence of these type of questions. It is always better to be prepared for these type of questions to land on the safer side.
There is no specific trick to solve these problems but some how we managed to create some shortcut tricks/methods to solve the question within 5 seconds. The best we can do is to create the most appropriate equation according to the question. Now, to create the equation out of the question we must ascertain knowledge on framing equation. Below given is the basic knowledge required.
Introduction
Practice these 10 Questions and it is done for Problem on Age section
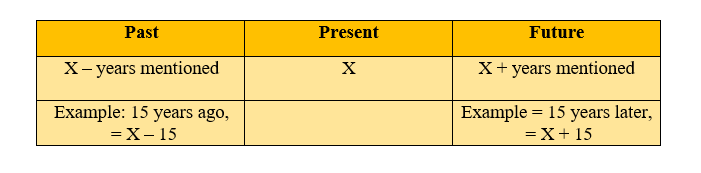
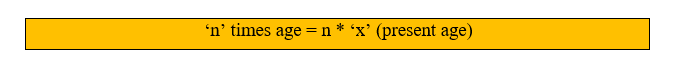

If you keep this above mentioned knowledge in fingertips then every problem on ages will be an easy one.
Note – Also keep in mind the difference between the age of two person is always same in past , present and future.
Basic Problem on Age Questions
Example 1 ) Steve’s age after 15 years will be 5 times his age 5 years back. What is the present age of Steve?
Solution :
Step 1 : Present age = X
Step 2 : Future age = X + 15 (Steve’s age after 15 years)
Step 3 : Past age = X – 5 (His age 5 years back)
Now, read the question 15 years will be 5 times his age 5 years back
So, Future Age ( X + 15) = 5 * (Past Age [ X-5])
=> X + 15 = 5 ( X – 5 )
=> X + 15 = 5 X – 25
=> 4 X = 40
=> X = 10.
The present age of Steve is 10.
Example 2 ) Jesvin is two years older than Anjana, who is twice as old as Diya. The total ages of Jesvin, Anjana and Diya be 27. How old is Anjana?
Solution :
Let us denote Jesvin age with ‘J’, Anjana age with ‘A’ and Diya age with ‘D’.
=> J = 2 + A (Jesvin is two years older than Anjana)–(1)
=> A = 2 D (Anjana is twice as old as Diya)–(2)
=> J + A + D = 27 (Total ages of Jesvin, Anjana and Diya be 27) — (3)
Substitute (1) & (2) in (3)
=> 2 + A + 2 D + D = 27
=> 2 + 2 D + 2 D + D = 27
=> 5 D = 27 – 2
=> 5 D = 25
=> D = 5
So, Diya’s age is 5.
The question demands the age of Anjana.
Then, A = 2 D (Check equation number 2)
So, A = 2*5
So, A = 10.
The age of Anjana is 10.
The answer is 10.
Example 3 ) The present age of Sumesh and Naresh is in the ratio 5:4 ratio respectively. Three years hence, their ages will become 11:9 respectively. What is Naresh’s present age?
Solution :
Note – Hence means after
Let us denote Sumesh age by ‘S’ and Naresh age by ‘N’
S = 5 X — (1)
N = 4 X — (2)
5 X + 3 11
________ = ______
4 X + 3 9
( + 3 is added because the question says three years hence which denotes the future. So 3 is added both to Sumesh and Naresh. Because after 3 years both of them will get old. So, 3 is added to both of them.)
Cross multiply,
45 X + 27 = 44 X + 33
X = 6.
Substitute X = 6 in (2) to find out the present age of Naresh.
(2) = 6 X
=> 6 * 4
= 24 years.
The age of Naresh is 24 years.
The answer is 24 years.
Example 4 – Padmini is 40 years old Ramya is 60 years old. How many years ago was the ratio of their ages 3:5?
Solution :
Lets us suppose “X” years ago their ratio is 3:5 , so their ages X year ago will be
Padmini’s age as 40 – X [ present age – X ]
Ramya’s age as 60 – X [ present age – X ]
=> 40 – X 3
_______ = ______
60 – X 5
Cross multiply the equation
=> ( 40 – X ) 5 = 3 ( 60 – X )
=> 200 – 5 X = 180 – 3 X
=> 20 = 2 X
X = 10 Years.
The answer is 10 years. So 10 years ago their ratio of ages is 3:5.
Note – A shortcut trick is also explained below to solve this question of age ratio in 10 seconds
Example 5 ) Peter’s present age is 2/5 th of his mother. After 8 years, he will be one-half of the age of his present mother. How old is the mother at present?
Solution :
Let X be the age of Peter
Let M be the age of Peter’s mother.
=> X = 2/5 (M) – Peter’s present age is 2/5 th of his mother
=> M = 5X / 2 ———– (1)
After 8 years, Peter’s age increases by 8 years and also his mom’s age increases by 8 years.

Advance Level Questions
Example 6 ) The sum of the present ages of father and his son is 60 years. 6 years ago, the father’s age was five times the age of the son. After 6 years, the son’s age will be?
Let us denote Son’s present age as ‘X’
Let us denote Father’s present age as ‘Y’
=> X + Y = 60 (Sum of present ages of father and son is 60)
=> Y = 60 – X (Taking X to the Right-Handed Side) —- (1)
Six years ago, the father’s age was five times the age of the son.
=> ( Y – 6) = 5 ( X- 6) —— (2)
Substitute (1) in (2); Substitute Y as 60 – X
=> 60 – X – 6 = 5 ( X – 6 )
Multiply 5 inside the bracket in Right-Handed Side.
=> 60 – X – 6 = 5 X – 30
=> 54 – X = 5 X – 30
Bring – X to R.H.S and – 30 to L.H.S
=> 54 + 30 = 5 X + X
=> 84 = 6 X or 6 X = 84
=> X = 14
The present age of Son is 14.
The question is what is the age of son after 6 years?
Then, add 6 to 14
The age of Son after 6 years will be 20.
The answer is 20.
Example 7 ) A man has 2 sons. Presently, the man’s age is thrice the sum of the ages of his 2 sons. Five years hence, the father’s age will be twice the sum of the ages of his son’s. What is the age of the father?
Let the age of father be denoted by ‘X’
Let the age of the two sons be denoted as ‘Y and Z’
Presently, the age of the man is thrice the sum of the ages of his two sons.
=> X = 3 ( Y + Z ) ———– ( 1 )
Five years hence, the father’s age will be twice the sum of the ages of his sons , So after 5 years –
Father age = X + 5
1st Son age = Y + 5
2nd Son age = Z + 5
=> X + 5 = 2 ( Y + 5 + Z + 5) — ( 2 ) [Adding 5 on the both the side of ( 1 )
Sub ( 1 ) in ( 2 )
=> 3 Y + Z + 5 = 2 Y + 10 + 2 Z + 10
=> 3 Y – 2 Y + 5 = 10 + 2 Z – Z + 10 (Bring 2Y in L.H.S and Z in R.H.S)
=> Y + 5 = 10 + Z + 10
=> Z + Y = 15 — (3)
Substitute ( 3 ) in ( 1 )
=> X = 3 ( Y + Z )
=> X = 3 ( 15 )
=> X = 45
The present age of father is 45
Example 8 ) Consider 5 siblings born apart by 3 years each. If the sum of all the ages of the children is 50 years. What is the age of the youngest child?
There is a gap of three years between each sibling
Let us consider that X is the youngest child.
Then add 3 years to each child.
The sum of all ages is 50.
( X ) + ( X + 3 ) + ( X + 6 ) + ( X + 9 ) + ( X + 12 ) = 50.
X + X + 3 + X + 6 + X + 9 + X +12 = 50
5 X + 30 = 50 [ Add all the X and numbers in L.H.S ]
5 X = 50 – 30 (Bring 30 in R.H.S)
5 X = 20
X = 20/5
X = 4
The youngest child is denoted by X
The age of the youngest sibling is 4.
Trick to solve the age ratio questions in 10 second
Yet, there is one trick to solve ratio problems within a span of 10 seconds. We will get you there step by step. Concentrate and you will achieve it easily.
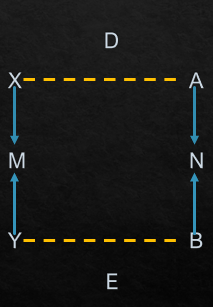
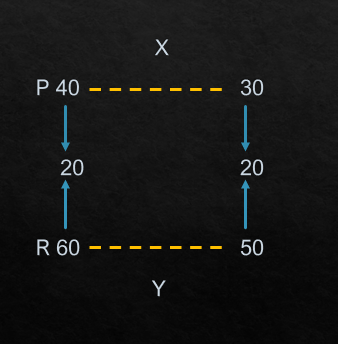
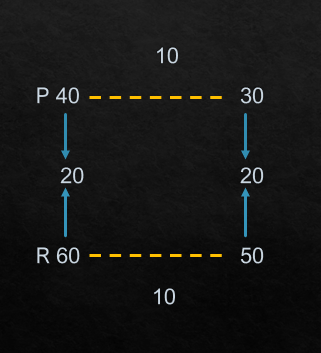
Yeah, I know you are unable to interpret the picture shown above.
Explanation of diagram
1) X and Y are ages of the particular person.
2) A and B are the ages of the same persons after some time(years).
3) D and E is the time travel from age X -> Y and A -> B . D and E will always be same.
4) M is the positive age difference of X and Y [M = X-Y ].
5) N is the positive age difference of A and B [N = A-B ].
6) M and N always be positive , if difference comes negative then change it sign to make it positive.
Steps to Solve question
1) X,Y,A ,B ,D,E are all given in question.
2) Find M and N as explained above where M = X-Y and N =A-B.
3) Now minimize the M and N by dividing by each other , If they are equal and then go to step 5 , else multiple A and B with M and X and Y with N [after minimizing them].
4) Now calculate again M and N , now they will be equal .
5) Now check X – A = D or not , if X-A not equal to D then multiply the numbers X , Y , A and B with D.
6) Now after making X-A=D now , and M and N also equal , now we have the answers.
7) X ,Y,A,B are all real age now and D is the travel time of age from X to A.
Note – It looks complex but it is very easy once you understand this logic.
Let’s solve some examples.
Example 1 ) The ratio of P and Q is 5 : 8. Three years later their ages will be in the ration 8 : 11. What is the present age of Q ?

Now here
X = present age of P and Y = present age of Q , A = P’s age after 3 years and B = P’s age after 3 years.
1) X = 5 , Y = 8 , A = 8 , B=11 given
2) calculate M = X-A = -3 but we need to take positive only so make it 3 , N = Y – B = 3.
3) now M and N are already equal.
4) D and E is given time travel from X to A = 3 years
5) D and E are also equal .
6) Now M =N and D =E are both equal , so X , Y , A , B are all real age.
7) Y is the present of Q = 8 years.
Let us take the other example No.4 mentioned above.
Example 2 )Padmini is 40 years old Ramya is 60 years old. How many years ago was the ratio of their ages 3:5?
Solution – Traditional Method
Let us denote Padmini’s age as 40 X
Let us denote Ramya’s age as 60 X
=> 40 – X 3
_______ = ______
60 – X 5
Cross multiply the equation
=> ( 40 – X ) 5 = 3 ( 60 – X )
=> 200 – 5 X = 180 – 3 X
=> 20 = 2 X
X = 10 Years.
The answer is 10 years.
Now, let us show you the Padhle way of solving this sum in an easier and wiser manner.
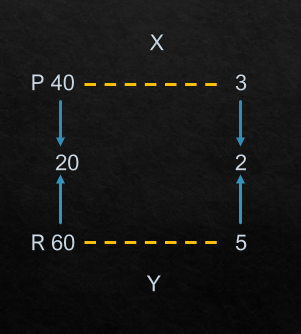
This time, there are two changes.
1. The difference of M and N is not equal.
2. We need to find the travel time i.e. X and Y ( as mentioned in the picture ).
Step 1: Make M and N equal. Do it with cross-multiplication. For that minimize them by dividing each other with any number if it it can and then do cross multiplication like shown below or explained above.
Take LCM between M and N and do cross-multiplication as shown below.

Now the following is the equation which will be derived from the above multiplication.
Now, the equation has become equal.
Now M = N and X-A = 10 which is time travel.
Can you notice how much the time could have been taken to travel years ago to get this age.
I heard you correct, it is 10.
10 years ago their ratio was 3 : 5.
The answer is 10 years.
Just practice few more questions like this you will become pro in this method.
below is the video to understand this trick in better way
Practice some previous year asked questions of Problem on Ages
Some More tricks using option method
There are other tricks to solve certain questions of ages.
For example,
Example 1) A & B ages are in the ratio 4:5. Four year hence, their age ratio becomes 5:6. What is B’s present age?
a) 20 years
b) 16 years
c) 24 years
d) Data insufficient
e) None of these.
Solution:
The question is to find the present age of B. So, see the present ratio of A and B which is 4:5. So, B’s ratio is 5.
Find the multiples of 5 in options. So, the answer here is 20.
Note -the present year ratio is always the multiple of present age.
Lets take one more similar example – This question solved above also with another trick.- , so if option method works then go with option method first.
Example 2 ) The ratio of present ages of P and Q is 5 : 8. Three years later their ages will be in ratio 8 : 11. What is the present age (in years) of Q?
Options:
1) 5
2) 11
3) 14
4) 8
Solution – we have to find present age of Q , the ratio of present age of Q is 8 , so present age must be the multiple of 8 , check the options for multiple of 8 and there is only one multiple of 8 that is 8 , so the present age of Q is 8.
Note – This is not applicable to all questions as we may have answers where we have more than two multiple of present age.
Even with multiple you can find answers in seconds.
Let us see another example,
Ques ) The present age of father is five times the ages of his son. Five years ago, the age of father was ten times the age of his son at that time. How old is father at present?
a) 45 years
b) 40 years
c) 48 years
d) 59 years
e) None of these
Solution:
Let us see the first option,
a) 45 years
As per the first statement the age of father is 5 times the age of son. Then, the age of son must be 9. Because 5*9 = 45.
As per the second statement: 5 years ago, the age of father was ten times the age of son. Then, the age of father 5 years ago is 45-5 = 40. If father’s age is decreased then the age of son is also decreased. Then, the age of son 5 years ago is 9-5 = 4. Now, is father’s age ten times the age of son? Yes, the son’s age is 4 and father’s age is 40. (4*10=40).
The answer is 45.
Note – You have to cross verify if all the statements do match before you opt an option.
If you have any doubts then you are free to post your queries in the comment section.