Compound Interest Tricks , Formulae and Questions

Compound interest calculates interest on both the initial principal and the accumulated interest from previous periods. The question based on interest calculation has frequently appeared in the many question papers of government exams and placement papers. The formulae are given below.

$1. CI = A - P$

$2. A = P( 1 + \frac{R}{100})^T$

Difference between Simple Interest and Compound Interest

Calculating Simple Interest and Compound interest for Rs 100 at 20 % p.a

Simple Interest	Compound Interest
Principal = 100 1st year Interest 10% of 100 = Rs 10	Principal = 100 1st year Interest 10% of 100 = Rs 10 Amount = P + I = 100 + 10 = 110
Principal = 100 2nd year Interest 10% of 100 = Rs 10	Principal = Amount after 1st year=110 2nd year Interest 10% of 110 = Rs 11 Amount = P + I = 110 + 11 = 121
Total Interest 1st Year Interest + 2nd Year Interest = 10 + 10 = 20	Total Interest 1st Year Interest + 2nd Year Interest = 10 + 11 = 21
Total Amount after 2nd year 100 + 10 + 10 = 120	Total Amount after 2nd year 100 + 10 + 11 = 121

Note –
* In compound interest , Interest is always calculated on the Amount of previous year.
* Compound Interest is always greater than Simple interest if interest rate is same and applied for same period of time.

The above example is understandable enough to solve questions.

Important note:
We can solve the maximum compound interest question without using the formulae as shown in the above example. By finding the values and then work it out as the formula results in power. Finding power without a calculator is a hard task.
1. Use formula when the Rate of interest is not given.
2. Don’t use formula when the Rate of interest is given.

There is a special formula to calculate the interest of the compound interest.

$Interest = Interest_1 + Interest_2 + \frac{Interest_1 * Interest_2}{100}$

$Interest_1 = Interest-percent-of-the-first-year$

$Interest_2 = Interest-percent-of-the-second-year$

$Amount = Principal + Principal * Interest$

Condition: This formula is applicable only for two successive interest rates.

We will solve without using the formula and also by using the special formula mentioned above. The participant can adopt whichever finds easier.

Let’s solve some questions.
Question 1 ) Find the amount due on Rs 8000 in 2 years if the rate of compound interest is 10% for the first year and 12% for the second year.
A) Rs 9716 B) Rs 9856 C) Rs 10156 D) Rs 9756

Solution: The Rate of interest is given. Let’s solve this problem as shown in the example.

Principal =8000
1st Year – Interest Rate – 10 %
Interest = ( 10 % of 8000 ) = 800
Amount at the end of 1st Year = 8000 + 800 = 8800

2nd year
Principal = Amount at the end of 1st Year = 8800
2nd Year – Interest Rate – 12 %
Interest = ( 12 % of 8800 ) = 1056
Amount at the end of 2nd Year = 8800 + 1056= 9856

The amount is Rs. 9856

[OR]

Let’s calculate this by the special trick formula.

$Interest = I_1 + I_2 + \frac{I_1*I_2}{100}$

Here,
I1 = Interest of the first year = 10
I2 = Interest of the second year = 12
Let’s substitute I1 & I2 in the special trick

$Interest = 10+12 + \frac{10*12}{100}$

$Interest = 22 + \frac{120}{100} = 22+1.2 = 23.2$

Interest = 23.2%

$Amount = Principal + Principal * Interest$

$Amount = 8000 + 8000 * \frac{23.2}{100} = 8000 + 1856 = 9856$

Answer: B

Question 2 ) The compound interest on Rs. 30,000 at 7% p.a. is Rs. 4347. The period (in years) is _.
A) 3 years B) 4 years C) 2 years D) 1 year

Solution: Rate of Interest is given and so we need not use formula.

Principal = 30,000
1st Year – Interest Rate – 7 %
Interest = ( 7 % of 30000) = 2100
Amount at the end of 1st Year = 30000+ 2100= 32100

2nd year
Principal = Amount at the end of 1st Year = 32100
2nd Year – Interest Rate – 7 %
Interest = ( 7 % of 32100 ) = 2247
Amount at the end of 2nd Year = 32100+ 2247= 34347

The question asks in how many years will 30,000 at 7% p.a. will result at the amount 34347. It took two years to arrive at 34347.

Answer:C

Important note:
If any interest is compounded semi-annually then we need to divide the interest rate by 2 and find the amount twice for one year.
For quarterly, the interest rate has to be divided by 4 and then the amount will be calculated 4 times for one year on the preceding value.
The above statements will be more clear if the below image is checked.

Difference between Annualy , Semi Anually / Half Yearly and Quarterly

Annually	Half Yearly Semi – Annually	Quarterly
Interest is calculated once a year.	Interest is calculated after every 6 months of time. Interest is calculated once in a half year [ 6 month ]. Interest is calculated 2 times in a year.	Interest is calculated after very 3 months on time. Interest is calculated once in a quarter [ 3 month ]. Interest is calculated 4 times in a year.

Lets understand with some examples

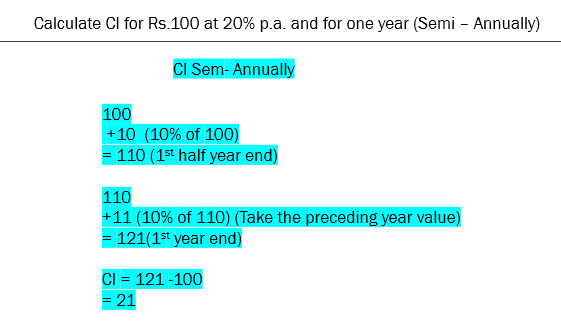
Compound Interest Semi anually — **The interest rate of 20% is divided into two parts of 10%. The first part of 10% is calculated in first year half and the remaining in the second half.**

Compound interest Quarterly — ***Here the 20% is divided by 4 and we are using 5% four times in order to one year itself.***

Question 3 ) What will Rs. 2000 amount to in two years if it is invested in 20% p.a. compound interest, interest being compounded semiannually?
A) Rs. 2880 B) Rs. 3160 C) Rs. 2928.20 D) Rs. 3148.40

Solution: Since R is given, we won’t use formula.

Compound Interest Semi Annually — ***The first year 20% is divided among the first year. The second year 20% is divided in the second year***

We can divide the 20% of one year into two parts for each year.
1st year 20% into two parts of 10% and 10%.
2nd year 20% into two parts of 10% and 10%.

$Interest = I_1 + I_2 + \frac{I_1*I_2}{100}$

$Interest = 10 + 10 + \frac{10*10}{100} = 10 + 10 + 1 = 21$

First year interest = 21%. As we know second year interest is divided into two parts of 10 & 10. We need to add till the last 10% gets added as shown below.

$Interest = 21+10+\frac{21*10}{100} = 21 + 10 + 2.1 = 33.1$

Interest at the end of two and a half year

$Interest = 33.1 + 10 + \frac{33.1*10}{100} = 33.1 + 10 + 3.31 = 46.41$

Total interest = 46.41 %

$Amount = Principal + Principal * Interest$

$Amount = 2000 + 2000 * \frac{46.41}{100} = 2000 + 928.2 = 2928.2$

Answer: C

Question 4 ) Find the compound interest (reckoned yearly) on Rs. 2400 at 10% p.a. for 2 years 4 months.
A) Rs. 3000.80 B) Rs. 400.80 C) Rs. 600.80 D) Rs. 700

Solution: R is given and so no use of formula.

Answer: C

Question 5 ) The difference between CI & SI on a certain sum at 10% p.a. for 2 years in Rs.631. Find sum.
A. 63,100 B. 6,310 C. 63200 D. 63,000

Solution: Here we are using a trick to solve this question in a easy manner , just try to focus and understand it.
Let’s find the SI and CI for 10% p.a. for 2 years on any sample amount. Let us consider the sample amount of Rs. 100.

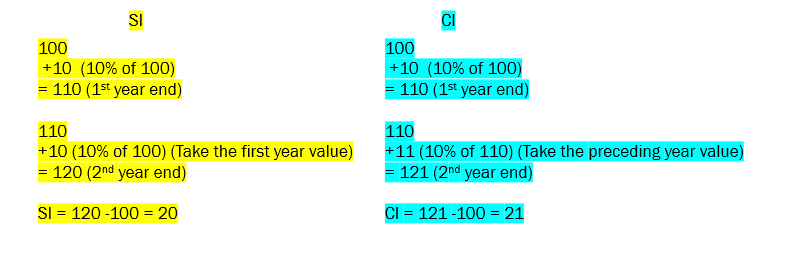
For the sample amount the difference amount is 1 for 10% p.a. and for 2 years.

Question 6) If the amount becomes 6 ¼ times of the principal after 2 years of CI, the rate of interest p.a. is
A) 115% B) 150% C) 15% D) 105%

Solution: The R is not given in the question. So, we gotta use the CI formula in order to solve the formula.
As per given, 6 1/4 can also be written as 6.25.
A = 6.25 P (The amount becomes 6 1/4 times of the principal)
Given, T = 2. P and R is not given.

$P (1 + \frac{R}{100}) ^2 = 6.25 P$

P gets cancelled
6.25 can also be written as

$\frac{625}{100}$

$(1 + \frac{R}{100}) ^2 = \frac {625}{100}$

( 625 = 25 square and 100 = 10 square )

$( 1+ \frac{R}{100})^2 = (\frac{25}{10})^2$

The square on both the sides gets cancelled

$1 + \frac{R}{100} = \frac{25}{10}$

$\frac{R}{100} = \frac{25}{10} - 1$

$\frac{R}{100} = \frac{15}{10}$

$R = 150%$

Answer = B

Question 7) There is 60% increase in an amount in 6 years at simple interest. What will be the compound interest on Rs. 12,000 after 3 years at the same rate?
A) Rs. 2160 B) Rs. 3120 C) Rs. 3972 D) Rs. 6240

Solution: Given R = 60 % for 6 years, then how much is for one year?

$R =\frac{60}{6} = 10$